-
Controlling the systematic uncertainties is critical for obtaining meaningful results in lattice QCD. For example, the nonperturbative renormalization method of the Rome-Southampton collaboration [1] has been widely used to convert from the lattice scheme to continuum schemes, avoiding the introduction of errors from the slowly converging lattice perturbation theory. Another example is the use of Symanzik improvement [2, 3] to systematically reduce discretization errors due to nonzero lattice spacing a. Since it is crucial to understand the mixing patterns of the operators involved, understanding the symmetries of a problem provides a powerful nonperturbative method. Symmetries could protect certain mixings from happening, while those not protected by symmetries could occur under quantum corrections. Although symmetry considerations do not provide a quantitative analysis of the mixing, they do provide a complete mixing pattern among operators in the problem.
In this work, we use the symmetries of lattice QCD to analyze the mixing pattern of a class of nonlocal quark bilinear operators defined in Eq. (27). Their renormalization in the continuum has been discussed since the 1980s [4, 5]. In recent years, there has been renewed interest in the renormalization of these operators in the context of methods for calculating the Bjorken-x dependence of the hadron parton distribution functions (PDFs) using the lattice QCD, the quasi-PDF method [6] and its variations [7, 8]. For recent progress in this area, see Refs. [9–56]. A special feature of these nonlocal quark bilinears is that the Wilson line connecting the quark fields receives power-divergent contributions. A nonperturbative subtraction of the power divergence was proposed in Refs. [33, 34, 57] by recasting the Wilson line as a heavy quark field in the auxiliary field approach [4, 5] such that the counterterm needed to subtract the power divergence is just the counterterm for heavy quark mass renormalization. The renormalization of the nonlocal quark bilinears in the continuum was studied in Refs. [19, 35, 36] , on a lattice in Ref. [27], and in nonperturbative renormalization schemes in [14, 15].
A lattice theory has fewer symmetries than its corresponding continuum theory. This implies that there will be more mixing among the operators in a lattice theory than in the corresponding continuum theory. For example, a pioneering one-loop lattice perturbation theory calculation using Wilson fermions showed that the breaking of chiral symmetry for the Wilson fermions induces the mixing shown in Eq. (34) [26]. In this work, instead of performing explicit computations, we use symmetries to systematically study the mixing patterns among nonlocal quark bilinears (part of this work was reported in [15, 58]). We study not only the mixing among the lowest dimensional nonlocal quark bilinears of mass dimension-three as was done in Ref. [26], but also the mixing between dimension-three and dimension-four operators, which cannot be avoided even if chiral symmetry is preserved①. This feature is confirmed by the computation of an example one-loop diagram.
Our study is particularly relevant to the quasi-PDF approach, which receives power corrections in inverse powers of hadron momentum. It is important to find the window where hadron momentum is large enough to suppress power corrections (good progress was made using momentum smearing in [13, 59]), but small enough that mixing with dimension-four operators is under control. In the following, we first review the symmetry analysis of local quark bilinear operators, and then move to the nonlocal ones.
-
If the
$ \theta $ term is neglected, the lattice action exhibits important discrete symmetries: the action is invariant under discrete parity ($ {\cal P} $ ), time reversal ($ {\cal T} $ ) and charge conjugation ($ {\cal C} $ ) transformations (see e.g. Ref. [60]). Chiral symmetry, which is a continuous symmetry, however, might be broken after the fermion fields are discretized. In this section, we review the symmetry properties for a specific set of local quark bilinear operators under these transformations. We then extend the analysis to nonlocal quark bilinear operators in the next section. The importance of these analyses is that if two operators transform differently, then the symmetries will protect them from mixing with each other under quantum corrections to all orders in the coupling. Operators not protected from mixing by the symmetries will, in general, mix. -
In this subsection, we summarize the transformations of fields under
$ {\cal P} $ ,$ {\cal T} $ ,$ {\cal C} $ and the axial transformation (the vector transformation in chiral symmetry is conserved for all operators that we study). We work in the Euclidean spacetime with coordinates$ (x, y, z, \tau) = $ (1,2,3,4) throughout this paper. Gamma matrices are chosen to be Hermitian:$ \gamma_{\mu}^{\dagger} = \gamma_{\mu} $ , and$ \gamma_5 = \gamma_1\gamma_2\gamma_3\gamma_4 $ .Since there is no distinction between time and space in the Euclidean space, the parity transformation, denoted
$ {\cal P}_{\mu} $ with$ \mu\in\{1,2,3,4\} $ , can be defined with respect to any direction.$ \psi(x)\xrightarrow[]{{\cal P}_{\mu}} \psi(x)^{{\cal P}_{\mu}} = \gamma_{\mu}\psi(\mathbb{P}_{\mu}(x)), $

(1) $ \overline{\psi}(x)\xrightarrow[]{{\cal P}_{\mu}} \overline{\psi}(x)^{{\cal P}_{\mu}} = \overline{\psi}(\mathbb{P}_{\mu}(x))\gamma_{\mu}, $

(2) $ U_{\nu\not = \mu}(x)\xrightarrow[]{{\cal P}_{\mu}} U_{\nu\not = \mu}(x)^{{\cal P}_{\mu}} = U_{\nu\not = \mu}^{\dagger}(\mathbb{P}_{\mu}(x)-\hat{\nu}), $

(3) $ U_{\mu}(x)\xrightarrow[]{{\cal P}_{\mu}} U_{\mu}(x)^{{\cal P}_{\mu}} = U_{\mu}(\mathbb{P}_{\mu}(x)), $

(4) where
$ \mathbb{P}_{\mu}(x) $ is the vector x with the flipped sign, except for the$ \mu $ -direction.Similarly, the time reversal transformation, denoted as
$ {\cal T}_{\mu} $ , can be generalized in any direction in the Euclidean space.$ \psi(x)\xrightarrow[]{{\cal T}_{\mu}} \psi(x)^{{\cal T}_{\mu}} = \gamma_{\mu}\gamma_5\psi(\mathbb{T}_{\mu}(x)), $

(5) $ \overline{\psi}(x)\xrightarrow[]{{\cal T}_{\mu}} \overline{\psi}(x)^{{\cal T}_{\mu}} = \overline{\psi}(\mathbb{T}_{\mu}(x))\gamma_5\gamma_{\mu}, $

(6) $ U_{\mu}(x)\xrightarrow[]{{\cal T}_{\mu}} U_{\mu}(x)^{{\cal T}_{\mu}} = U_{\mu}^{\dagger}(\mathbb{T}_{\mu}(x)-\hat{\mu}), $

(7) $ U_{\nu\not = \mu}(x)\xrightarrow[]{{\cal T}_{\mu}} U_{\nu\not = \mu}(x)^{{\cal T}_{\mu}} = U_{\nu\not = \mu}(\mathbb{T}_{\mu}(x)), $

(8) where
$ \mathbb{T}_{\mu}(x) $ is the vector x with the flipped sign in the$ \mu $ -direction.Charge conjugation
$ {\cal C} $ transforms particles into antiparticles,$ \psi(x)\xrightarrow[]{\cal C} \psi(x)^{\cal C} = C^{-1}\overline{\psi}(x)^{\top}, $

(9) $ \overline{\psi}(x)\xrightarrow[]{\cal C} \overline{\psi}(x)^{\cal C} = -\psi(x)^{\top}C, $

(10) $ U_{\mu}(x)\xrightarrow[]{\cal C} U_{\mu}(x)^{\cal C} = U_{\mu}(x)^{\ast} = (U_{\mu}^{\dagger}(x))^{\top}, $

(11) and
$ C\gamma_{\mu}C^{-1} = -\gamma_{\mu}^{\top}, \qquad C\gamma_5C^{-1} = \gamma_5^{\top}. $

(12) The continuous axial rotation (
$ {\cal A} $ ) of the fermion fields is$ \psi(x)\xrightarrow[]{\cal{A}}\psi'(x) = {\rm e}^{{\rm i}\alpha\gamma_5}\psi(x),\quad \overline{\psi}(x)\xrightarrow[]{\cal{A}}\overline{\psi}'(x) = \overline{\psi}(x){\rm e}^{{\rm i}\alpha\gamma_5}, $

(13) where
$ \alpha $ is the x-independent rotation angle of the global transformation②. The explicit axial symmetry breaking pattern induced by the quark mass m can be studied by introducing a spurious transformation$ m\xrightarrow[]{\cal{A}}{\rm e}^{-{\rm i}\alpha\gamma_5}m{\rm e}^{-{\rm i}\alpha\gamma_5}, $

(14) so that the quark mass term is invariant under this extended axial transformation.
-
We now study the transformation properties of a class of local quark bilinear operators of the form
$ O_{\Gamma} = \overline{\psi}(x)\Gamma\psi(x), $

(15) with
$ \Gamma\in\{{\bf{1}}, \; \gamma_{\mu}, \; \gamma_{\bf{5}}, \; {\bf{i}}\gamma_{\mu}\gamma_{\bf{5}}, \; \sigma_{\mu\nu}\}, $

(16) where
$ \sigma_{\mu\nu} = \frac{i}{2}[\gamma_{\mu},\gamma_{\nu}] $ . Quantum loop effects for these operators are in powers of$ \log a $ . The Hermitian conjugate is$ (O_{\Gamma})^{\dagger} = -O_{\gamma_4\Gamma\gamma_4} = -G_4(\Gamma)O_{\Gamma}, $

(17) where
$ G_{\mu}(\Gamma) $ , which has a value of either$ +1 $ or$ -1 $ , satisfies$ \gamma_{\mu}\Gamma\gamma_{\mu} = G_{\mu}(\Gamma)\Gamma. $

(18) Therefore, depending on
$ \Gamma $ , the expectation value of$ O_{\Gamma} $ can be purely real or imaginary.Under
$ {\cal P} $ ,$ {\cal T} $ , and$ {\cal C} $ , the local quark bilinear transforms as$ O_{\Gamma}\xrightarrow[]{{\cal P}_{\mu}} O_{\gamma_{\mu}\Gamma\gamma_{\mu}},\;\;\; O_{\Gamma}\xrightarrow[]{{\cal T}_{\mu}} O_{\gamma_5\gamma_{\mu}\Gamma\gamma_{\mu}\gamma_5},\;\;\; O_{\Gamma}\xrightarrow[]{\cal C} O_{(C\Gamma C^{-1})^{\top}}. $

(19) $ O_{\Gamma} $ either stays invariant (even, E) or changes sign (odd, O) under a transformation. The results are summarized in Table 1. Operators of different$ \Gamma $ do not mix under renormalization, since they transform differently under$ {\cal P}_{\mu} $ or$ {\cal T}_{\mu} $ .$ {\cal C} $ alone does not protect the operators from mixing with each other.$\Gamma=\bf{1}$ 

$\gamma_{\mu}$ 

$\gamma_5$ 

$i\gamma_{\mu}\gamma_5$ 

$\sigma_{\mu\nu}$ 

${\cal P}_{\rho=\mu}$ 

E E O O O ${\cal P}_{\rho\not=\mu}$ 

E O O E O ${}_{(\rho=\nu)}$ /E

${}_{(\rho\not=\nu)}$ 

${\cal T}_{\rho=\mu}$ 

E O O E O ${\cal T}_{\rho\not=\mu}$ 

E E O O O ${}_{(\rho=\nu)}$ /E

${}_{(\rho\not=\nu)}$ 

${\cal C}$ 

E O E E O ${\cal A}$ 

V I V I V Table 1. Properties of the dimension-three local operator
$O_{\Gamma}$ under parity (${\cal P}_{\rho}$ ), time reversal (${\cal T}_{\rho}$ ), charge conjugation (${\cal C}$ ) and axial (${\cal A}$ ) transformations. E and O stand for even and odd, while I and V stand for invariant and variant under transformations.Under an axial rotation (with Eq. (14) included),
$ O_{\Gamma} $ is either invariant (I) or variant (V), as shown in Table 1. Some lattice fermions, such as Wilson fermions, break the axial symmetry, but from the above discussion we see that axial symmetry is not essential for protecting$ O_{\Gamma} $ from mixing. Only$ {\cal P}_{\mu} $ or$ {\cal T}_{\mu} $ is needed. -
For dimension-four, we can further classify the operators into p type and m type operators, which have one more insertion of derivative or quark mass, respectively, compared with the dimension-three operators. Here p denotes a typical momentum in the external state. It is useful to define the covariant derivatives,
$ \overrightarrow{D}_{\mu} $ and$ \overleftarrow{D}_{\mu} $ , acting on a field$ \phi(x) $ ,$ \overrightarrow{D}_{\mu}\phi(x) = \frac{1}{2a}\left[U_{\mu}(x)\phi(x+\hat{\mu}a) -U_{\mu}^{\dagger}(x-\hat{\mu}a)\phi(x-\hat{\mu}a)\right], $

(20) $ \phi(x)\overleftarrow{D}_{\mu} = \frac{1}{2a}\left[\phi(x+\hat{\mu}a)U_{\mu}^{\dagger}(x) -\phi(x-\hat{\mu}a)U_{\mu}(x-\hat{\mu}a)\right]. $

(21) The Euclidean four-dimensional rotational symmetry dictates that p type operators are constructed by inserting
$ \overrightarrow{\not \!\!\!D} $ and$ \overleftarrow{\not\!\!\! D} $ into$ O_{\Gamma} $ :$ Q_{\Gamma\!\overrightarrow{D}} = \overline{\psi}(x)\Gamma\overrightarrow{\not\!\!\! D}\psi(x),\qquad Q_{\overleftarrow{D}\!\Gamma} = \overline{\psi}(x)\overleftarrow{\not\!\!\! D}\Gamma\psi(x), $

(22) $ Q_{\overrightarrow{D}\!\Gamma} = \overline{\psi}(x)\overrightarrow{\not\!\!\! D}\Gamma\psi(x),\qquad Q_{\Gamma\!\overleftarrow{D}} = \overline{\psi}(x)\Gamma\overleftarrow{\not\!\!\! D}\psi(x). $

(23) It can be shown that these operators transform in the same way as
$ O_{\Gamma} $ under$ {\cal P}_{\mu} $ and$ {\cal T}_{\mu} $ , while under$ {\cal C} $ ,$ \begin{split} &Q_{\Gamma\!\overrightarrow{D}/\overrightarrow{D}\!\Gamma} \xrightarrow[]{\cal C} -Q_{\overleftarrow{D}\!(C\Gamma C^{-1})^{\top} /(C\Gamma C^{-1})^{\top}\!\overleftarrow{D}},\\\qquad &Q_{\overleftarrow{D}\!\Gamma/\Gamma\!\overleftarrow{D}} \xrightarrow[]{\cal C} -Q_{(C\Gamma C^{-1})^{\top}\!\overrightarrow{D} /\overrightarrow{D}\!(C\Gamma C^{-1})^{\top}}, \end{split} $

(24) with the operators
$ \overrightarrow{\not\!\!\! D} $ and$ \overleftarrow{\not \!\!\!D} $ transforming into each other. Therefore, it is convenient to define the combinations$ O_{\Gamma}^{p(\pm)} = Q_{\overleftarrow{D}\!\Gamma}\pm Q_{\Gamma\!\overrightarrow{D}},\qquad O_{\overline{\Gamma}}^{p(\pm)} = Q_{\Gamma\!\overleftarrow{D}}\pm Q_{\overrightarrow{D}\!\Gamma}, $

(25) which are either even or odd under
$ {\cal C} $ . The transformation properties of the p type operators are listed in Table 2. By comparing with Table 1, we observe that$ {\cal P}_{\mu} $ ,$ {\cal T}_{\mu} $ and$ {\cal C} $ symmetries do not protect$ O_{\Gamma} $ from mixing with$ O_{\Gamma/\overline{\Gamma}}^{p(-)} $ , but the axial symmetry does. So, if the lattice theory preserves axial or chiral symmetry, then the dimension-three and p type dimension-four operators studied above will not mix.$\Gamma=\bf{1}$ 

$\gamma_{\mu}$ 

$\gamma_5$ 

$i\gamma_{\mu}\gamma_5$ 

$\sigma_{\mu\nu}$ 

${\cal P}_{\rho=\mu}$ 

E E O O O ${\cal P}_{\rho\not=\mu}$ 

E O O E O ${}_{(\rho=\nu)}$ /E

${}_{(\rho\not=\nu)}$ 

${\cal T}_{\rho=\mu}$ 

E O O E O ${\cal T}_{\rho\not=\mu}$ 

E E O O O ${}_{(\rho=\nu)}$ /E

${}_{(\rho\not=\nu)}$ 

${\cal C}(O_{\Gamma/\overline{\Gamma}}^{p(+)})$ 

O E O O E ${\cal C}(O_{\Gamma/\overline{\Gamma}}^{p(-)})$ 

E O E E O ${\cal A}$ 

I V I V I Table 2. Transformation properties of the dimension-four
$p$ type local operators$O_{\Gamma/\overline{\Gamma}}^{p(\pm)}$ . Notation is the same as in Table 1.We now consider the m type operators. The only operator that appears at this order is
$ O_{\Gamma}^{m} = m\overline{\psi}(x)\Gamma\psi(x), $

(26) which transforms in the same way as
$ O_{\Gamma} $ under$ {\cal P}_{\mu} $ ,$ {\cal T}_{\mu} $ and$ {\cal C} $ . However, it transforms differently from$ O_{\Gamma} $ under$ {\cal A} $ .Therefore, we conclude that if the lattice theory preserves axial or chiral symmetry, then the dimension-three and dimension-four operators (including both the p type and m type operators) studied above will not mix.
-
Having reviewed the operator-mixing properties of the local quark bilinears, we now apply the analysis to a specific type of nonlocal quark bilinears.
-
We are interested in the nonlocal quark bilinear operators with quark fields separated by
$ \delta z $ in the z-direction:$ O_{\Gamma}(\delta z) = \overline{\psi}(x+\delta z)\Gamma U_3(x+\delta z;x)\psi(x), $

(27) where a straight Wilson line
$ U_3 $ is added such that the operators are gauge invariant. Treating the z-direction differently from the other directions, we write$ \Gamma\in\{{\bf{1}}, \; \gamma_{i}, \; \gamma_{3}, \; \gamma_{5}, \; {i}\gamma_{i}\gamma_{5}, \; {i}\gamma_{3}\gamma_{5}, \; \sigma_{i3}, \; \epsilon_{ijk}\sigma_{jk}\}, $

(28) where
$ i, j, k\not = 3 $ . These operators receive quantum loop corrections as powers of$ 1/a $ and$ \log a $ [19, 35, 36]. It is important to keep in mind that one cannot take the continuum limit of the matrix elements of these operators.Under
$ {\cal P}_{\mu} $ and$ {\cal T}_{\mu} $ ,$ O_{\Gamma}(\delta z)\xrightarrow[]{{\cal P}_{l\not = 3}} O_{\gamma_l\Gamma\gamma_l}(-\delta z),\quad O_{\Gamma}(\delta z)\xrightarrow[]{{\cal P}_3} O_{\gamma_3\Gamma\gamma_3}(\delta z), $

(29) $ O_{\Gamma}(\delta z)\xrightarrow[]{{\cal T}_{l\not = 3}} O_{\gamma_5\gamma_l\Gamma\gamma_l\gamma_5}(\delta z),\quad O_{\Gamma}(\delta z)\xrightarrow[]{{\cal T}_3} O_{\gamma_5\gamma_3\Gamma\gamma_3\gamma_5}(-\delta z). $

(30) The transformations could change the sign of
$ \delta z $ , so it is convenient to define$ O_{\Gamma\pm}(\delta z) = \frac{1}{2}\left(O_{\Gamma}(\delta z)\pm O_{\Gamma}(-\delta z)\right), $

(31) whose Hermitian conjugate yields
$ (O_{\Gamma\pm}(\delta z))^{\dagger} = \mp G_4(\Gamma)O_{\Gamma\pm}(\delta z). $

(32) Thus, the expectation value of
$ O_{\Gamma\pm}(\delta z) $ is either purely real or purely imaginary, depending on$ \Gamma $ . Under$ \cal C $ ,$ O_{\Gamma\pm}(\delta z) \xrightarrow[]{\cal C}\pm O_{(C\Gamma C^{-1})^{\top}\pm}(\delta z). $

(33) The transformation properties of
$ O_{\Gamma\pm}(\delta z) $ are listed in Table 3. We see that$ {\cal P}_{\mu} $ ,$ {\cal T}_{\mu} $ and$ {\cal C} $ symmetries cannot protect the mixing between$ {\bf{1}} $ and$ \gamma_3 $ or between$ i\gamma_i\gamma_5 $ and$ \epsilon_{ijk}\sigma_{jk} $ operators of dimension-three. This can be summarized as$\Gamma=\bf{1}_{+/-}$ 

$\gamma_{i+/-}$ 

$\gamma_{3+/-}$ 

$\gamma_{5+/-}$ 

$i\gamma_i\gamma_{5+/-}$ 

$i\gamma_3\gamma_{5+/-}$ 

$\sigma_{i3+/-}$ 

$\epsilon_{ijk}\sigma_{jk+/-}$ 

${\cal P}_3$ 

E O E O E O O E ${\cal P}_{l\not=3}$ 

E/O E/O $_{(l=i)}$ 

O/E O/E O/E $_{(l=i)}$ 

E/O O/E $_{(l=i)}$ 

E/O $_{(l=i)}$ 

O/E $_{(l\not=i)}$ 

E/O $_{(l\not=i)}$ 

E/O $_{(l\not=i)}$ 

O/E $_{(l\not=i)}$ 

${\cal T}_3$ 

E/O E/O O/E O/E O/E E/O O/E E/O ${\cal T}_{l\not=3}$ 

E O $_{(l=i)}$ 

E O E $_{(l=i)}$ 

O O $_{(l=i)}$ 

E $_{(l=i)}$ 

E $_{(l\not=i)}$ 

O $_{(l\not=i)}$ 

E $_{(l\not=i)}$ 

O $_{(l\not=i)}$ 

${\cal C}$ 

E/O O/E O/E E/O E/O E/O O/E O/E ${\cal A}$ 

V I I V I I V V Table 3. Transformation properties of the dimension-three nonlocal operators
$O_{\Gamma\pm}(\delta z)$ .$i,j,k\not=3$ . Other notation is the same as in Table 1.$ O_{\Gamma\pm}(\delta z)\xrightarrow[]{{\rm{mixes \ with}}} (1+G_3(\Gamma))O_{\gamma_3\Gamma\mp}(\delta z), $

(34) which is consistent with the mixing pattern found using the lattice perturbation theory in Refs. [26, 27]. However, if the lattice theory preserves axial or chiral symmetry, then none of the dimension-three operators will mix with each other.
The mixing among dimension-three operators of different
$ \delta z $ cannot be excluded by symmetries, but diagrammatic analysis excludes this possibility to all orders in the strong coupling constant expansion [35]. The mixing of dimension-three with dimension-four operators of different$ \delta z $ has not been systematically studied yet. However, the one-loop example in Eq. (48) is consistent with no mixing among operators of different$ \delta z $ . -
We now extend the discussion for p type and m type local operators to nonlocal ones. We can insert
$ \not\!\!{D} $ at any point on the Wilson line. The symmetry properties will not depend on where$ \not\!\!{D}$ is inserted.$ \begin{split} Q_{\Gamma\!\overrightarrow{D}_{\alpha}}(\delta z,\delta z') = & \overline{\psi}(x+\hat{3}\delta z) U_3(x+\hat{3}\delta z;x+\hat{3}\delta z')\\&\times\Gamma\overrightarrow{\not \!\!\!D}_{\alpha} U_3(x+\hat{3}\delta z';x) \psi(x), \end{split}$

(35) $ \begin{split} Q_{\overrightarrow{D}_{\alpha}\Gamma}(\delta z,\delta z') = & \overline{\psi}(x+\hat{3}\delta z) U_3(x+\hat{3}\delta z;x+\hat{3}\delta z')\\&\times\overrightarrow{\not \!\!\!D}_{\alpha}\Gamma U_3(x+\hat{3}\delta z';x) \psi(x), \end{split}$

(36) $ \begin{split} Q_{\Gamma\!\overleftarrow{D}_{\alpha}}(\delta z,\delta z') = & \overline{\psi}(x+\hat{3}\delta z) U_3(x+\hat{3}\delta z;x+\hat{3}\delta z') \\&\times\Gamma \overleftarrow{\not\!\!\! D}_{\alpha}U_3(x+\hat{3}\delta z';x)\psi(x), \end{split}$

(37) $ \begin{split} Q_{\overleftarrow{D}_{\alpha}\Gamma}(\delta z,\delta z') = & \overline{\psi}(x+\hat{3}\delta z)U_3(x+\hat{3}\delta z;x+\hat{3}\delta z')\\&\times\overleftarrow{\not \!\!\!D}_{\alpha} \Gamma U_3(x+\hat{3}\delta z';x)\psi(x), \end{split}$

(38) where
$ 0\leqslant \delta z' \leqslant \delta z $ . The z-direction is treated differently by writing$ \alpha\in[3,\perp] $ and$ \overrightarrow{\not\!\!\! D}_3 = \gamma_3\overrightarrow{D}_3 $ , and$ \overrightarrow{\not \!\!\!D}_{\perp} = \sum_{\mu\not = 3}\gamma_{\mu}\overrightarrow{D}_{\mu} $ .As in the local quark bilinear case, inserting
$ \overrightarrow{\not\!\!\! D} $ and$ \overleftarrow{\not\!\!\!D} $ does not change the transformation properties under$ {\cal P}_{\mu} $ and$ {\cal T}_{\mu} $ . These operators transform in the same way as$ O_{\Gamma}(\delta z) $ . It is useful to define combinations that are even or odd under$ {\cal P}_{\mu} $ and$ {\cal T}_{\mu} $ :$ \begin{split} Q_{\Gamma\!\overrightarrow{D}_{\alpha}\pm/\overrightarrow{D}_{\alpha}\Gamma\pm}(\delta z,\delta z') =& \frac{1}{2}\left( Q_{\Gamma\!\overrightarrow{D}_{\alpha}/\overrightarrow{D}_{\alpha}\Gamma}(\delta z,\delta z')\right.\\&\left.\pm Q_{\Gamma\!\overrightarrow{D}_{\alpha}/\overrightarrow{D}_{\alpha}\Gamma}(-\delta z,-\delta z') \right), \end{split}$

(39) $ \begin{split} Q_{\Gamma\!\overleftarrow{D}_{\alpha}\pm/\overleftarrow{D}_{\alpha}\Gamma\pm}(\delta z,\delta z') = & \frac{1}{2}\left( Q_{\Gamma\!\overleftarrow{D}_{\alpha}/\overleftarrow{D}_{\alpha}\Gamma} (\delta z,\delta z')\right.\\&\left.\pm Q_{\Gamma\!\overleftarrow{D}_{\alpha}/\overleftarrow{D}_{\alpha}\Gamma} (-\delta z,-\delta z') \right). \end{split}$

(40) Under
$ \cal C $ , these operators transform as$ Q_{\Gamma\!\overrightarrow{D}_{\alpha}\pm/\overrightarrow{D}_{\alpha}\Gamma\pm}(\delta z,\delta z') \xrightarrow[]{\cal C} \mp Q_{\overleftarrow{D}_{\alpha}(C\Gamma C^{-1})^{\top}\pm /(C\Gamma C^{-1})^{\top}\!\overleftarrow{D}_{\alpha}\pm}(\delta z,\delta z'), $

(41) $ Q_{\overleftarrow{D}_{\alpha}\Gamma\pm/\Gamma\!\overleftarrow{D}_{\alpha}\pm}(\delta z,\delta z') \xrightarrow[]{\cal C} \mp O_{(C\Gamma C^{-1})^{\top}\!\overrightarrow{D}_{\alpha}\pm /\overrightarrow{D}_{\alpha}(C\Gamma C^{-1})^{\top}\pm}(\delta z,\delta z'). $

(42) Hence, we define the combinations
$ Q_{\Gamma\pm/\overline{\Gamma}\pm}^{{D}_{\alpha}(+)}(\delta z,\delta z') = Q_{\overleftarrow{D}_{\alpha}\Gamma\pm/\Gamma\!\overleftarrow{D}_{\alpha}\pm}(\delta z,\delta z') +Q_{\Gamma\overrightarrow{D}_{\alpha}\pm/\overrightarrow{D}_{\alpha}\!\Gamma\pm}(\delta z,\delta z'), $

(43) $ Q_{\Gamma\pm/\overline{\Gamma}\pm}^{{D}_{\alpha}(-)}(\delta z,\delta z') = Q_{\overleftarrow{D}_{\alpha}\Gamma\pm/\Gamma\!\overleftarrow{D}_{\alpha}\pm}(\delta z,\delta z') -Q_{\Gamma\overrightarrow{D}_{\alpha}\pm/\overrightarrow{D}_{\alpha}\!\Gamma\pm}(\delta z,\delta z'), $

(44) such that
$ Q_{\Gamma\pm/\overline{\Gamma}\pm}^{{D}_{\alpha}(+)}(\delta z,\delta z') \xrightarrow[]{\cal C} \mp Q_{(C\Gamma C^{-1})^{\top}\pm/(C\overline{\Gamma}C^{-1})^{\top}\pm}^{{D}_{\alpha}(+)}(\delta z,\delta z'), $

(45) $ Q_{\Gamma\pm/\overline{\Gamma}\pm}^{{D}_{\alpha}(-)}(\delta z,\delta z') \xrightarrow[]{\cal C} \pm Q_{(C\Gamma C^{-1})^{\top}\pm/(C\overline{\Gamma}C^{-1})^{\top}\pm}^{{D}_{\alpha}(-)}(\delta z,\delta z'). $

(46) Their properties under
$ {\cal P}_{\mu} $ ,$ {\cal T}_{\mu} $ and$ {\cal C} $ are listed in Table 4. Comparing with Table 3, we find that$ {\cal P}_{\mu} $ ,$ {\cal T}_{\mu} $ and$ {\cal C} $ symmetries do not protect$ O_{\Gamma}(\delta z) $ from mixing with$ Q_{\Gamma}^{{D}_{\alpha}}(\delta z,\delta z') $ or$ Q_{\gamma_3\Gamma}^{{D}_{\alpha}}(\delta z,\delta z') $ . If the lattice theory preserves axial or chiral symmetry, then the mixing with$ Q_{\Gamma}^{{D}_{\alpha}}(\delta z,\delta z') $ is forbidden, but the mixing with$ Q_{\gamma_3\Gamma}^{{D}_{\alpha}}(\delta z,\delta z') $ is still allowed. Since the Wilson line can be described as a heavy quark propagator in the auxiliary field approach [19, 27, 35], this is analogous to the static heavy-light system, which has p type discretization errors even if the light quarks respect chiral symmetry. Note that Ref. [58] did not include the operators with$ \delta z' $ different from$ 0 $ and$ \delta z $ . Since there are many more p type operators now, it makes the nonperturbative improvement program advocated in Ref. [58] much more difficult, and perhaps impractical.$\Gamma=\bf{1}_{+/-}$ 

$\gamma_{i+/-}$ 

$\gamma_{3+/-}$ 

$\gamma_{5+/-}$ 

$\gamma_i\gamma_{5+/-}$ 

$\gamma_3\gamma_{5+/-}$ 

$\sigma_{i3+/-}$ 

$\epsilon_{ijk}\sigma_{jk+/-}$ 

${\cal P}_3$ 

E O E O E O O E ${\cal P}_{l\not=3}$ 

E/O E/O $_{(l=i)}$ 

O/E O/E O/E $_{(l=i)}$ 

E/O O/E $_{(l=i)}$ 

E/O $_{(l=i)}$ 

O/E $_{(l\not=i)}$ 

E/O $_{(l\not=i)}$ 

E/O $_{(l\not=i)}$ 

O/E $_{(l\not=i)}$ 

${\cal T}_3$ 

E/O E/O O/E O/E O/E E/O O/E E/O ${\cal T}_{l\not=3}$ 

E O $_{(i=l)}$ 

E O E $_{(l=i)}$ 

O O $_{(l=i)}$ 

E $_{(l=i)}$ 

E $_{(l\not=i)}$ 

O $_{(l\not=i)}$ 

E $_{(l\not=i)}$ 

O $_{(l\not=i)}$ 

${\cal C}$ (

$Q_{\Gamma\pm/\overline{\Gamma}\pm}^{{D}_{\alpha}(+)}$ )

O/E E/O E/O O/E O/E O/E E/O E/O ${\cal C}$ (

$Q_{\Gamma\pm/\overline{\Gamma}\pm}^{{D}_{\alpha}(-)}$ )

E/O O/E O/E E/O E/O E/O O/E O/E $\cal A$ 

I V V I V V I I Table 4. Transformation properties of the dimension-four
$p$ type nonlocal operators$Q_{\Gamma\pm/\overline{\Gamma}\pm}^{{D}_{\alpha}(\pm)}(\delta z,\delta z')$ .$i,j,k\not=3$ . Other notation is the same as in Tab. 1.The m type nonlocal bilinear is
$ Q_{\Gamma}^{\rm M}(\delta z) = m\overline{\psi}(x+\hat{\bf 3}\delta z)\Gamma U_3(x+\hat{\bf 3}\delta z;x)\psi(x). $

(47) It has the same transformation properties as
$ O_{\Gamma}(\delta z) $ under$ {\cal P}_{\mu} $ ,$ {\cal T}_{\mu} $ and$ {\cal C} $ but is different for the chiral rotation. However, chiral symmetry does not prevent$ O_{\Gamma}(\delta z) $ from mixing with the m type operator$ Q_{\gamma_3\Gamma}^{\rm M}(\delta z) $ . -
In the previous section, it was shown that
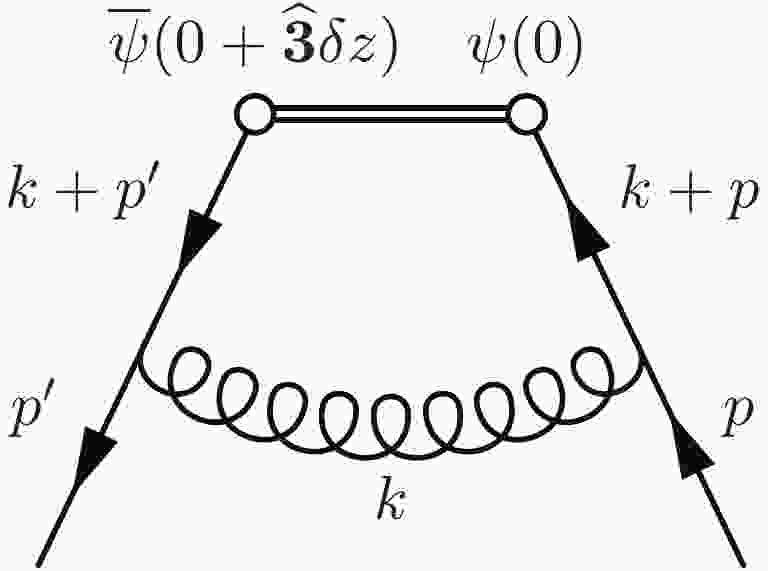
$ {\cal P}_{\mu} $ ,$ {\cal T}_{\mu} $ ,$ {\cal C} $ , and chiral symmetries cannot protect dimension-three nonlocal quark bilinears from mixing with dimension-four operators. This is a distinct feature, different from local quark bilinears in which dimension-three operators are protected from mixing with dimension-four operators. Here, we use the diagram shown in Fig. 1 to demonstrate where the effect comes from. For our purpose, we can simplify the calculation by taking the Feynman gauge and the limit of small external momenta and quark masses, and we work in the continuum limit with appropriate UV and IR regulators imposed implicitly. The one-loop amputated Green function in Fig. 1,$ \Lambda_{\Gamma, \delta z}^{\rm{1-loop}}(p', p, m) $ , then yields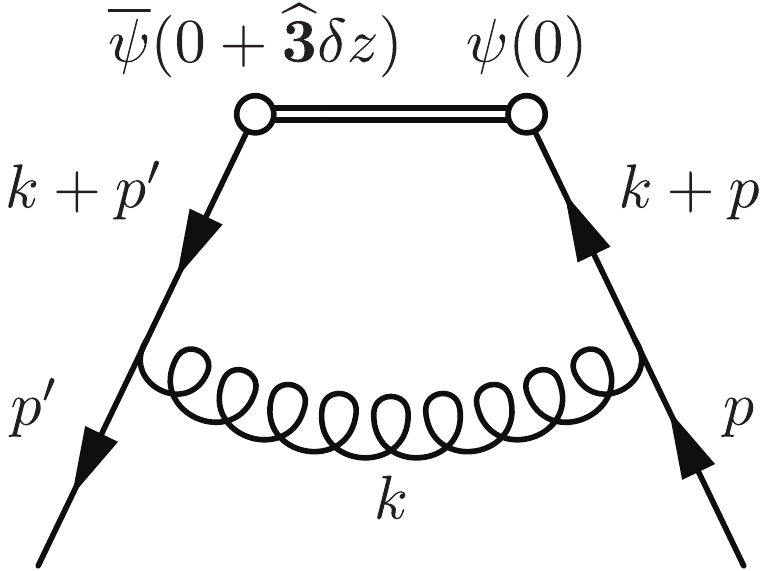
Figure 1. One of the one-loop Feynman diagrams for the nonlocal quark bilinear.
$p$ and$p'$ are incoming and outgoing external momenta, respectively.$ \begin{split}\Lambda_{\Gamma, \delta z}^{\rm{1-loop}}& (p', p, m) {\rm e}^{{\rm i}p_3'\delta z}\!=\! \Gamma \!+\!\!\int_k\frac{\delta_{\mu\nu}\delta_{AB}}{k^2}(-ig\gamma_{\mu}T^A)\\& \times\frac{1}{i({\not k}\!+\!{\not\!\! p}')\!+\!m} \Gamma {\rm e}^{-{\rm i}k_3\delta z}\frac{1}{i({\not k}+{\not \!\!p})+m} (-ig\gamma_{\nu}T^B) \\ = & \left(1+g^2G_F{\cal A}_{\Gamma, \delta z}^{}\right) \Gamma +g^2G_F{\cal A}_{\Gamma, \delta z}^{m}(1+G_3(\Gamma))m\gamma_3\Gamma \\ &+g^2G_F{\cal A}_{\Gamma, \delta z}^{p_3} i\left\{ (1+G_3(\Gamma))(-{\not\!\! p}_3'\gamma_3\Gamma-\gamma_3\Gamma{\not \!\!p}_3) \right.\\&\left.+(1-G_3(\Gamma))(-{\not\!\! p}_3'\gamma_3\Gamma+\gamma_3\Gamma{\not\!\! p}_3) \right\} \\ &+g^2G_F{\cal A}_{\Gamma, \delta z}^{p_{\perp}} i\left\{ (1+G_3(\Gamma)) (-{\not\!\! p}_{\perp}'\gamma_3\Gamma-\gamma_3\Gamma{\not\!\! p}_{\perp}) \right.\\&\left.+(1-G_3(\Gamma)) (-{\not\!\! p}_{\perp}'\gamma_3\Gamma+\gamma_3\Gamma{\not\!\! p}_{\perp}) \right\} \\ &+g^2G_F{\cal A}_{\overline{\Gamma}, \delta z}^{p_{\perp}} i\left\{ (1+G_3(\Gamma)) (-\gamma_3\Gamma{\not\!\! p}_{\perp}'-{\not\!\! p}_{\perp}\gamma_3\Gamma)\right.\\&\left. +(1-G_3(\Gamma)) (-\gamma_3\Gamma{\not\!\! p}_{\perp}'+{\not\!\! p}_{\perp}\gamma_3\Gamma) \right\} \\ &+{\cal O}(p'^2, p^2, p'p, p'm, pm, m^2), \end{split} $

(48) where the coefficients are
$\begin{split} {\cal A}_{\Gamma, \delta z}^{} =& \int_k\frac{\cos(k_3\delta z)}{(k^2)^3} \frac{H(\Gamma)}{3}\left( \left(H(\Gamma)-G_3(\Gamma)\right)k^2\right.\\&\left. +\left(-H(\Gamma)+4G_3(\Gamma)\right)k_3^2 \right),\end{split} $

(49) $ {\cal A}_{\Gamma, \delta z}^{m} = \int_k\frac{\sin(k_3\delta z)k_3}{(k^2)^3} \left(-H(\Gamma)+2G_3(\Gamma)\right), $

(50) $\begin{split} {\cal A}_{\Gamma, \delta z}^{p_3} =& \int_k\frac{\sin(k_3\delta z)k_3}{(k^2)^4} \frac{H(\Gamma)}{6}\left(\left(-2H(\Gamma)+5G_3(\Gamma)\right)k^2 \right.\\&\left.+2\left(H(\Gamma)-4G_3(\Gamma)\right)k_3^2\right), \end{split}$

(51) $ {\cal A}_{\Gamma, \delta z}^{p_{\perp}} = \int_k\frac{\sin(k_3\delta z)k_3}{(k^2)^4} \frac{G_3(\Gamma)}{6}\left(\left(H(\Gamma)-6G_3(\Gamma)\right)k^2 +2H(\Gamma)k_3^2\right), $

(52) $ {\cal A}_{\overline{\Gamma}, \delta z}^{p_{\perp}} = \int_k\frac{\sin(k_3\delta z)k_3}{(k^2)^4} \frac{1}{3}\left(\left(-H(\Gamma)+3G_3(\Gamma)\right)k^2+H(\Gamma)k_3^2\right), $

(53) and where
$ H(\Gamma) = \sum_{\mu = 1}^4G_{\mu}(\Gamma) $ . It is easy to see that when$ \delta z = 0 $ (corresponding to a local quark bilinear), the mixings with all dimension-four operators vanish, but when$ \delta z \ne 0 $ (corresponding to a nonlocal quark bilinear), the mixing with dimension-four operators appears even though the theory has$ {\cal P} $ ,$ {\cal T} $ ,$ {\cal C} $ , and chiral symmetries. -
We have used the symmetry properties of nonlocal quark bilinear operators under parity, time reversal and chiral or axial transformations to study the possible mixing among these operators. Below, we summarize our findings.
1) If the lattice theory preserves chiral symmetry, then the dimension-three nonlocal quark bilinear operators
$ O_{\Gamma\pm}(\delta z) $ of Eq. (31) are protected from mixing with each other, but they are not protected from mixing with the dimension-four operators of Eqs. (43), (44) and (47) with all possible values of$ \delta z' $ satisfying$ 0\leqslant \delta z' \leqslant \delta z $ :$ \begin{split} O_{\Gamma}^{p}(\delta z,\delta z') =& (1+G_3(\Gamma))Q_{\gamma_3\Gamma}^{{D}_{\alpha}(-)}(\delta z,\delta z') \\& +(1-G_3(\Gamma))O_{\gamma_3\Gamma}^{{D}_{\alpha}(+)}(\delta z,\delta z'),\end{split} $

(54) $ \begin{split} O_{\overline{\Gamma}}^{p}(\delta z,\delta z') =& (1+G_3(\Gamma)) Q_{\overline{\gamma_3\Gamma}}^{{D}_{\alpha}(-)}(\delta z,\delta z') \\&+(1-G_3(\Gamma)) O_{\overline{\gamma_3\Gamma}}^{{D}_{\alpha}(+)}(\delta z,\delta z'), \end{split}$

(55) $ O_{\Gamma}^{m}(\delta z) = (1+G_3(\Gamma))Q_{\gamma_3\Gamma}^{\rm M}(\delta z), $

(56) where
$ G_{\mu} $ is defined in Eq. (18). This mixing pattern is confirmed by an example calculation for a one-loop diagram, as shown in Sec. 3.3. Since there are many operators in Eqs. (54)–(56), it is impractical to remove the mixing using the improvement procedure.2) If the lattice theory breaks chiral symmetry, then the dimension-three nonlocal quark bilinear
$ O_{\Gamma\pm}(\delta z) $ mixes with$ (1+G_3(\Gamma))O_{\gamma_3\Gamma\pm}(\delta z) . $

(57) The operator
$ O_{\Gamma\pm}(\delta z) $ not only mixes with all the operators in Eqs. (54)–(56), but also with$ Q_{\Gamma}^{{D}_{\alpha}(-)}(\delta z,\delta z') $ ,$ Q_{\overline{\Gamma}}^{{D}_{\alpha}(-)}(\delta z,\delta z') $ and$ Q_{\Gamma}^{\rm M}(\delta z) $ for all possible values of$ \delta z' $ satisfying$ 0\leqslant \delta z' \leqslant \delta z $ .This study is particularly relevant for the quasi-PDF approach, which receives power corrections in inverse powers of hadron momentum. It is important to find a window where hadron momentum is large enough to suppress power corrections, but at the same time the mixing with p type dimension-four operators is under control. For future work, in light of the similarity between the Wilson line and the heavy quark propagator, it would be valuable to apply techniques developed for the heavy quark effective field theory on the lattice [61, 62] and the associated treatments to improve lattice artifacts [63–68].
Symmetry properties of nonlocal quark bilinear operators on a Lattice (LP3 Collaboration)
- Received Date: 2019-05-01
- Accepted Date: 2019-07-09
- Available Online: 2019-10-01
Abstract: Using symmetry properties, we determine the mixing pattern of a class of nonlocal quark bilinear operators containing a straight Wilson line along a spatial direction. We confirm the previous study that mixing among the lowest dimensional operators, which have a mass dimension equal to three, can occur if chiral symmetry is broken in the lattice action. For higher dimensional operators, we find that the dimension-three operators will always mix with dimension-four operators, even if chiral symmetry is preserved. Also, the number of dimension-four operators involved in the mixing is large, and hence it is impractical to remove the mixing by the improvement procedure. Our result is important for determining the Bjorken-x dependence of the parton distribution functions using the quasi-distribution method on a Euclidean lattice. The requirement of using large hadron momentum in this approach makes the control of errors from dimension-four operators even more important.



 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF













 DownLoad:
DownLoad: